Is 10/13 A Rational Number

Rational Numbers Formula

Before learning rational numbers formulas, let us recall what are rational numbers. A rational number is a fraction whose numerator is an integer and the denominator is a non-zero integer. But all fractions are not rational numbers as a fraction may as well take its numerator and/or denominator to be an irrational number(s). Let us learn the rational numbers formulas in item in the adjacent section. The set up of rational numbers is denoted by 'Q' and it includes:

The set up of natural numbers, N
The gear up of whole numbers, W
The ready of integers, W
fractions of integers where the denominator is non zero.

What Is Rational Numbers Formula?

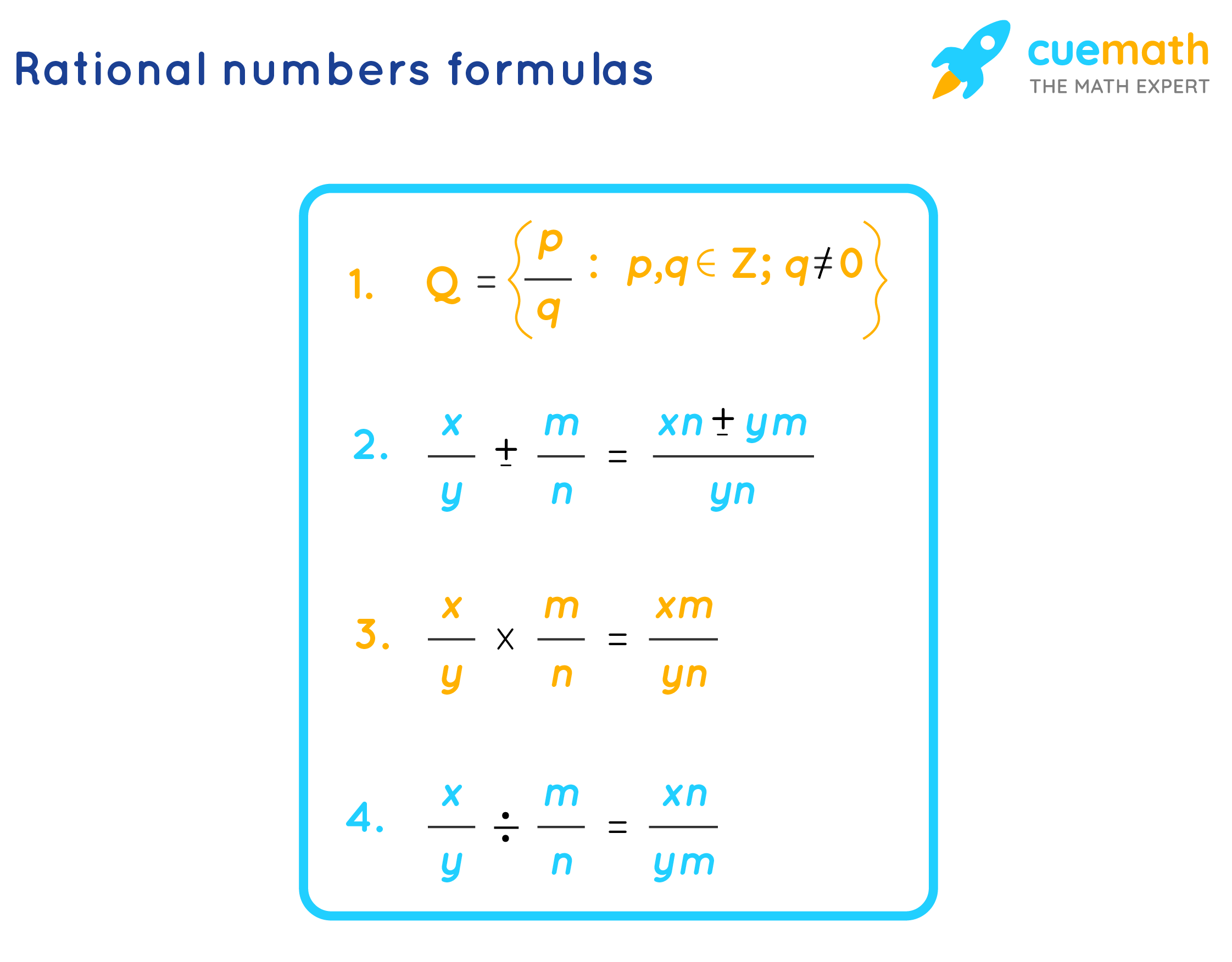
Using the definition of a rational number, which nosotros discussed in the previous section, a rational number is of the form \(\dfrac p q\), where 'p' and 'q' are integers and q≠0. Then some examples of rational numbers can be 2, -1, -3/2, 1/iii, 0, etc. We operate the rational numbers in just the way equally nosotros operate the fractions. Thus, the rational numbers formulas are:

\(\mathbb{Q} = \left\{\dfrac{p}{q} \,\,:\,\, p,q \in \mathbb{Z}; \,\, q\neq 0\right\}\)
\(\dfrac{x}{y} \pm \dfrac{yard}{n}=\dfrac{x n \pm y thousand}{y north}\)
\(\dfrac{ten}{y} \times \dfrac{yard}{n}=\dfrac{10 thou}{y north}\)
\(\dfrac{10}{y} \div \dfrac{m}{n}=\dfrac{ten n}{y m}\)

Annotation: The set of rational numbers is closed, associative, and commutative under addition and multiplication. The additive identity, 0, and the multiplicative identity, i are present in the set of rational numbers. All rational numbers have their additive inverses in the set of rational numbers. All rational numbers other than 0 have their multiplicative inverses in the set of rational numbers.

Rational numbers formulas

Breakdown tough concepts through simple visuals.

Math will no longer be a tough subject field, especially when y'all empathize the concepts through visualizations.

Volume a Gratuitous Trial Course

Let us see the usage of the rational numbers formulas in the following solved examples.

Example 1: Identify which of the following are rational numbers using the rational numbers formula: -2, \(\sqrt{two}\), \(\dfrac 1 2\), -\(\dfrac i 3\), and \(\dfrac {-1}{\sqrt ii}\).

Solution:

The given numbers can exist written equally:

-2 = \(\dfrac {-two} 1\), here both -ii and 1 are integers where one \(\neq \) 0.

\(\sqrt 2 \) = \(\dfrac {\sqrt 2} i\), merely here \(\sqrt ii\) is Not an integer.

\(\dfrac 1 ii\), here both ane and 2 are integers where 2 \(\neq \) 0.

-\(\dfrac 1 3\) = \(\dfrac {-ane} 3\), here both -1 and 3 are integers where iii \(\neq 0\).

\(\dfrac {-one}{\sqrt 2}\), though it is a fraction, \(\sqrt 2\) is NOT an integer.

Thus, only -2, \(\dfrac 1 two\), and -\(\dfrac one iii\) are rational numbers amidst the given numbers.

Answer: -two, \(\dfrac 1 2\), and -\(\dfrac 1 iii\) are rational numbers.

Example 2: Detect the sum, difference (in the given lodge), product, and the quotient (in the given order) of the following rational numbers: \(\dfrac one 3\) and \(\dfrac 2 v\).

Solution: We will detect the sum, difference, production, and the quotient using the rational numbers formulas.

\( \dfrac{1}{3}+ \dfrac{2}{5}= \dfrac{5}{15}+ \dfrac{six}{xv} = \dfrac{11}{15}\)

\( \dfrac{1}{3}- \dfrac{2}{5}= \dfrac{v}{15}- \dfrac{half-dozen}{15} = -\dfrac{1}{15}\)

\(\dfrac{1}{3}\times \dfrac{ii}{v}= \dfrac{one \times two}{3 \times 5} = \dfrac{2}{15}\)

\(\dfrac{one}{3}\div \dfrac{two}{five}= \dfrac{1}{iii} \times \dfrac{5}{ii} = \dfrac{5}{6}\)

Answer: Sum = \( \dfrac{11}{fifteen}\), Difference = \( -\dfrac{1}{15}\), Product = \( \dfrac{ii}{xv}\), and Quotient = \( \dfrac{five}{vi}\).

Example 3: Find five rational numbers betwixt 1 and ii?

Solution: When any two integers, are expressed in the form of p/q, where q ≠ 0, it is known as a rational number.

Let's get the solution step by step.

Allow's stand for 1 and ii every bit rational numbers:

1 can be written as x/x and 2 tin can be written as 20/10

Then,

The rational numbers between 10/10 and xx/10 or 1 and ii are {11/x, 12/10, xiii/10, 14/10, 15/ten, 16/ten, 17/ten, 18/10, 19/ten}

Answer: Hence, any five rational numbers betwixt 1 and 2 are xi/10, 12/x, 13/10, 14/x, and 15/ten.

FAQs on Rational Numbers Formula

What Are Rational Numbers And Rational Number Formulas?

A rational number is a number that is in the class of p/q, where p and q are integers, and q is not equal to 0. Examples of rational numbers include 1/two, 2/7, ane/9, 9/three, and and so on. The rational numbers formula applies to rational numbers. Rational numbers formulas are:

\(\mathbb{Q} = \left\{\dfrac{p}{q} \,\,:\,\, p,q \in \mathbb{Z}; \,\, q\neq 0\right\}\)
\(\dfrac{x}{y} \pm \dfrac{m}{n}=\dfrac{ten n \pm y m}{y n}\)
\(\dfrac{x}{y} \times \dfrac{grand}{due north}=\dfrac{ten m}{y northward}\)
\(\dfrac{ten}{y} \div \dfrac{grand}{north}=\dfrac{ten north}{y thousand}\)

What Is the Deviation Between Rational and Irrational Numbers?

A rational number is a number that tin can be expressed equally the ratio of two integers in the form p/q, where the denominator (q) should not be equal to zero. An irrational number cannot be expressed in the form of fractions. Rational numbers are terminating decimals whereas irrational numbers are non-terminating. An example of a rational number is xi/2, and Pi(π) which is equal to three.141592653589…….

Check If 0 a Rational Number Using Rational Numbers Formula?

Yes, 0 is a rational number considering it is an integer and it tin can be written in the grade: p/q = 0/1. Hence, 0 is a rational number. The rational numbers formula applies to 0.